| 1. |
If the number of sides in a polygon was doubled, the sum of its interior angles would increase by 1,260 . How many sides does the original polygon have? |
|
| 2. |
What is the number of sides in a regular polygon in which the measure of an interior angle is twelve more than six times the measure of an exterior angle? |
|
| 3. |
What is the measure of each interior angle in a regular hexagon?
| |  | | | 144 | |
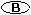| |  | | | 120 | |
| |  | | | 210 | |
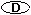| |  | | | 90 | |
| |  | | | 120 | |
|
|
| 4. |
How many diagonals can be drawn inside of a heptagon?
| | | | | 14 | |
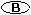| | | | | 1 | |
| | | | | 7 | |
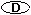| | | | | 13 | |
| | | | | None of the above | |
|
|
| 5. |
Polygon P has t sides. How many diagonals can be drawn inside of polygon P?
| | | | | 4 (t - 2) | |
| | | | | t(t - 3) ÷ 2 | |
| | | | | 2 (t) | |
| | | | | t(t - 2) ÷ 3 | |
| | | | | t(t - 2) ÷ 2 | |
| |  | | | t(t - 2) | |
|
|
| 6. |
If the sum of the measures of polygon is 2160, how many sides does the polygon have?
| | | | | 24 | |
| | | | | 17 | |
| | | | | 11 | |
| | | | | 20 | |
| | | | | 21 | |
| | | | | 14 | |
|
|
| 7. |
Each point of a polygon at which two sides intersect is called ___________.
| | | | | a side | |
| | | | | a vertex | |
| | | | | exterior angle | |
| | | | | interior angle | |
| | | | | diagonal | |
|
|
| 8. |
Polygon C has h sides. What is the sum of the measures of polygon's C interior angles?
| | | | | h(h - 3) ÷ 2 | |
| | | | | 180 (h - 2) ÷ 2 | |
| | | | | 360 (h - 2) | |
| | | | | 90 (h - 4) | |
| | | | | 180 (h - 2) | |
|
|
